Using mean-reversion in volatility to trade 0 DTE
So you degens want to indulge in 0dte, huh? Let's play.
A word of caution to begin with - this publication doesn't aim to become a 0 DTE specialist. There are people much more talented at that than we are, and we highly recommend you follow them.
However, due to popular demand, we decided to conduct another analysis on 0 DTE and try to find some strategies that may work. Moreover, 0 DTE has become such an important tool in the marketplace that one must understand how it functions. They're here to stay anyway. We've noticed with our Discord members the full list of daily contracts in IWM on Monday (you can now also trade options expiring on Tuesdays and Thursdays).
Another warning: We usually avoid 0 DTE, except when we have a well-quantified effect, like the overnight trade we presented a few weeks ago. We haven't started trading the findings in this article yet. However, considering how promising they look, we may start when the conditions are right.
That said, we hope you enjoy it, and if you do gamble, please do so responsibly. Considering the amount of gamma risk, these products are risky (we would argue much riskier than their longer-dated counterparts) and not worth losing your life savings over like a savage.
Finally, this is yet another piece of research: we do our best to make it reliable and give you some food for thought. It is certainly not financial advice.
Let's get started!
The curious case of Thursdays: the sequel.
A few months ago, we discussed the curious case of Thursdays. We showed that if you had to trade 0 DTE, you should follow Euan Sinclair's advice from his book "Positional Options Trading": the best day to sell them is Friday and exit on Monday. Alternatively, we found that selling on a Tuesday and exiting on Wednesday was also profitable.
More interestingly, we discovered that longing a straddle on Thursdays was often profitable. We stayed away from that trade as we couldn't find any good justification then. Sure, you could consider that the pre-market economic data on Thursday mornings justified it. However, back then, we had a hard time believing this. The market is much harder to beat than it looks, especially in the short term, and is not known to give away free lunches easily.
Was it a good decision?
It turns out it was. Almost immediately after we published this article in December 2023, long straddles stopped performing on Thursdays.
Seasonality exists in trading; however, it's often important to find a cause that can at least partially explain the phenomenon to avoid the correlation trap. The overnight trade in 0 DTE works well when VVIX is above 90 because the demand for hedges is higher than normal (as highlighted by the VVIX reading). It's unlikely that the supply will be as strong as it is when everything is calm, and there's no exacerbated demand for hedges.
For the rest of this study, we'll return to an important effect of options trading—the variance risk premium—and study its impact on the profitability of 0 DTE.
The curious VRP case for 0 DTEs.
Before we start, let’s outline the conditions of our research. We only looked into SPY, IWM, QQQ, and the daily contracts when available. We simulate the sale of a straddle at mid-price on a 1 DTE at 3:50 pm right before the close and an exit at 3:50 pm right before expiration. We also assume that each position is normalized to $1 of credit.
First, let's look at the naive strategy since 2022 and the introduction of daily contracts.
We can see that this strategy is overall profitable but doesn't seem to go anywhere in the case of SPY and QQQ. It's not a good idea to trade it, as the fees and execution error costs will most likely eat up all the profits.
IWM stands out, though. Why is that? It benefits again from the embedded premium inherent in the product's nature. Small and mid-cap companies are considered riskier than their blue-chip counterparts, and insurers often demand a greater premium.
When we saw the performance above, we immediately concluded that the variance risk premium drove this trade's profitability.
As a reminder, the Variance Risk Premium is defined as the ratio between the implied volatility and the realized volatility of the underlying. Options often imply a volatility level not realized in the underlying, offering option sellers a premium for the risk they take. That's why shorting straddles is, on average, profitable 59% of the time, with a slightly positive expectancy.
With IWM often having the highest implied volatility of the three, we can assume that it also has the highest VRP most of the time.
In this case, we directly compute the VRP using the 1 DTE straddle price and compare it to an average of 1 DTE moves observed recently in the underlying. A ratio above 1 indicates that the straddle is more expensive than the average of recent moves in the underlying, and vice versa.
We can see that the VRP is often higher in IWM, but it's not necessarily significantly bigger. Their VRPs are correlated over time, and nothing definitive can be concluded.
However, something caught our attention. When you plot the average return per straddle against the VRP, something counterintuitive happens.
The return per position is, on average, positive when... the VRP is below 1. Alternatively, it tends to be negative between 1 and 2. While IWM is somewhat immune to this observation, its return goes from almost 15% on average to just 1%.
QQQ, however, is barely profitable in the absence of VRP and loses a significant amount of money in its presence.
At this stage, it's important to note that our measure of the VRP, using an average of daily returns and making a ratio with the straddle price, is not perfect. However, it is still valid and avoids some serious complications.
Using annualized implied volatility and annual realized volatility on such small maturities is not ideal either (estimating implied volatility when the maturity is so close is notoriously problematic, particularly over weekends). If you find fundamentally different results, try reproducing them with the straddle prices.
Realized volatility is also mean-reverting (duh…)
At first, we thought these results might be due to anomalies in the dataset or that one-quarter of the data could significantly skew the rest. However, the results have been consistent over the past few years. They have been growing since the introduction of daily contracts for each day of the week and the constant increase in 0 DTE volume in the market.
So, how can we interpret these results? As a reminder, options trading is generally profitable in the presence of the VRP, not in its absence.
Due to the short-term nature of what we are currently measuring, there may be a simple explanation: the mean-reverting nature of realized volatility, particularly in the short term.
If the straddle price is lower than the average of 1 DTE moves recently observed in the underlying (VRP < 1), it may very well be that short-term realized volatility was extremely high recently and that we are overdue for a few calm and uneventful sessions.
As soon as you increase the number of DTEs and short, for instance, a 14 DTE straddle, the contrary is likely to happen: if things have been calm recently, they are likely to stay calm (a key characteristic of volatility is that it tends to cluster and be very similar to what you observed yesterday, in the absence of new information). Therefore, the presence of the VRP is a good time to sell.
Essentially, a longer timeframe averages out a couple of anomalies you may have observed throughout the week, allowing volatility sellers to make money when the VRP is present. On the other hand, on a super short-term timeframe, the mean-reverting nature of realized volatility plays against you: if things were extremely calm recently, so much so that the price of a straddle overstates the 1 DTE move, it is very likely that something will happen and derail your strategy, and vice versa.
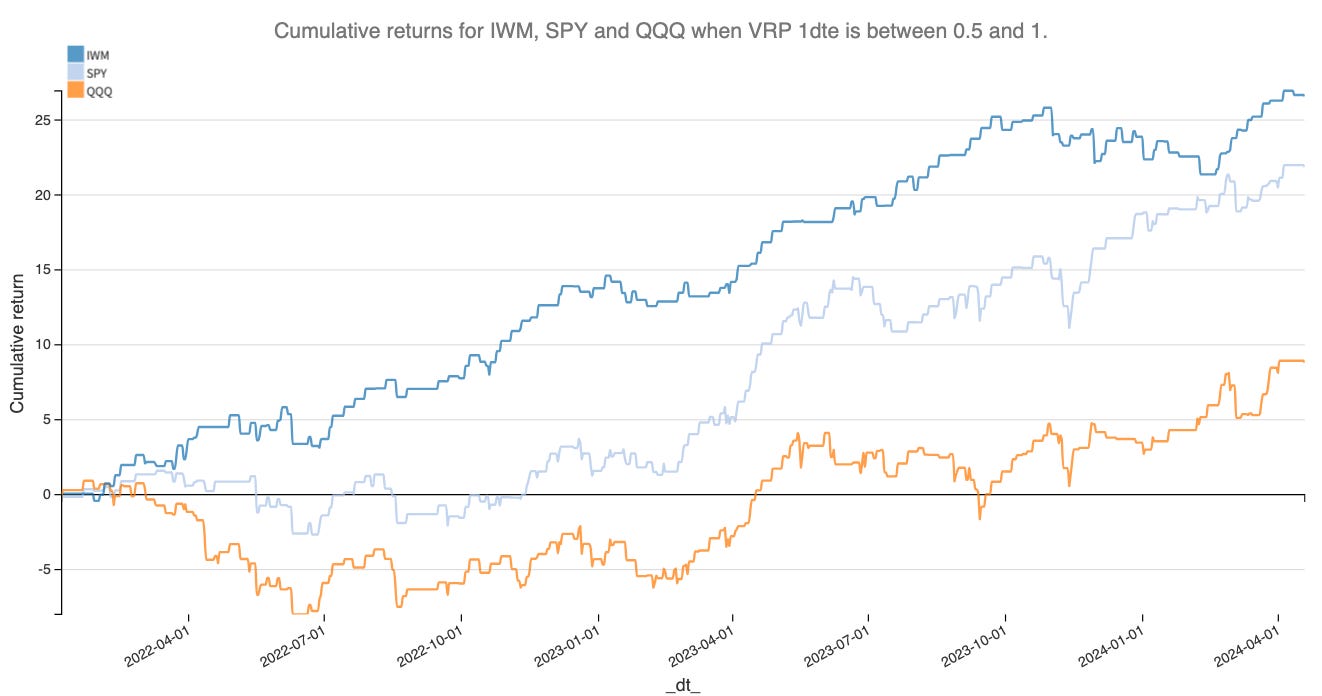
Let's look at the cumulative returns function when the VRP is between 0.5 and 1.
This is a significant improvement from the cumulative performance observed above. We take 50% fewer trades than the naive strategy and achieve a similar level of performance for IWM. However, SPY's performance doubles, while QQQ is still sluggish but turning positive.
We will write a follow-up article in a few months to see if these results hold. In the meantime, stay reasonable; trading is a long-term game, and this piece of research, if valid, is unlikely to be life-changing. However, adding it to your portfolio is a great way to stay active and add some diversification to the longer-term positions we usually talk about at Sharpe Two.
Data, charts, and analysis are powered by Thetadata and Dataiku DSS.
Have access to our indicators using our API.
Book a consultancy call to talk about the market with us.
Contact at info@sharpetwo.com.
Disclaimer: The information provided is solely informational and should not be considered financial advice. Before selling straddles, be aware that you risk the total loss of your investment. Our services might not be appropriate for every investor. We strongly recommend consulting with an independent financial advisor if you're uncertain about an investment's suitability.